Thermal Properties of Sea Water
Thermal Expansion. The coefficient of thermal expansion, e, defined by e = (l/αs,ϑ,p)(∂αs,ϑ,p/∂ϑ), is obtained, at atmospheric pressure, from the terms for D in Knudsen's Hydrographical Tables, and at higher pressures from Ekman's tables or formulae (p. 57). The coefficient for sea water is greater than that for pure water and increases with increasing pressure. A few numerical values are given in table 9, in which negative values indicate contraction with increasing temperature.
| Pressure (decibars) | Salinity ‰. | Temperature (°C) | |||||||
|---|---|---|---|---|---|---|---|---|---|
| −2 | 0 | 5 | 10 | 15 | 20 | 25 | 30 | ||
| 0………… | 0 | −105 | − 67 | 17 | 88 | 151 | 207 | 257 | 303 |
| 10 | − 65 | − 30 | 46 | 112 | 170 | 222 | 270 | 315 | |
| 20 | − 27 | 4 | 75 | 135 | 189 | 237 | 282 | 324 | |
| 30 | 7 | 36 | 101 | 157 | 206 | 250 | 292 | 332 | |
| 35 | 23 | 51 | 114 | 167 | 214 | 257 | 297 | 334 | |
| 2,000………… | 35 | 80 | 105 | 157 | 202 | 241 | 278 | ||
| 40 | 94 | 118 | 168 | 210 | 248 | 283 | |||
| 4,000………… | 35 | 132 | 152 | 196 | 233 | 266 | |||
| 40 | 144 | 162 | 204 | 240 | 272 | ||||
| 6,000………… | 34.85 | 177 | 194 | 230 | |||||
| 8,000………… | 34.85 | …… | 231 | 246 | |||||
| 10,000………… | 34.85 | …… | 276 | 287 | |||||
Thermal Conductivity. In water in which the temperature varies in space, heat is conducted from regions of higher to regions of lower
Specific Heat. The specific heat is the number of calories required to increase the temperature of 1 g of a substance 1°C. When studying liquids, the specific heat at constant pressure, cp, is the property usually measured, but in certain problems the specific heat at constant volume, cv must be known.
The specific heat of sea water of different chlorinities was investigated by Thoulet and Chevallier, whose results have been recalculated and presented in different ways. Krümmel (1907) gives the following values for the specific heat at 17.5°C and atmospheric pressure:
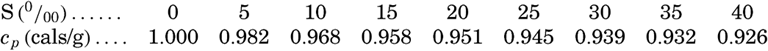
Kuwahara (1939) gives an empirical equation for the specific heat at 0°C and atmospheric pressure:
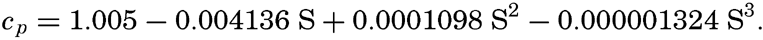
It will be noted that the specific heat decreases with increasing salinity, but it has been pointed out by Krümmel that the effect is somewhat larger than might be expected from the composition of the solution, and the problem merits reinvestigation. The effects of temperature and pressure have not been measured, but it has been assumed that they are the same as those for pure water. Ekman (1914) gives the following values showing the effect of temperature on the specific heat of water of S = 34.85 ‰ at atmospheric pressure:
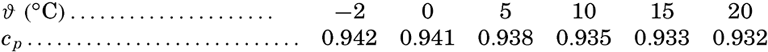
The effect of pressure on the specific heat has been computed by Ekman (1914) from the equation
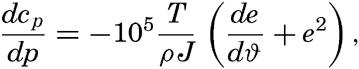
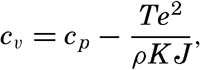
| θ (°C) | p (decibars) | ||||
|---|---|---|---|---|---|
| 2000 | 4000 | 6000 | 8000 | 10,000 | |
| − 2………… | 0.0171 | 0.0315 | 0.0435 | ||
| 0………… | 0.0159 | 0.0291 | 0.0401 | 0.0492 | 0.0566 |
| 5………… | 0.0136 | 0.0248 | 0.0340 | 0.0416 | 0.0479 |
| 10………… | 0.0120 | 0.0220 | |||
| 15………… | 0.0110 | 0.0203 | |||
| 20………… | 0.0105 | ||||
Latent Heat of Evaporation. The latent heat of evaporation of pure water is defined as the amount of heat in gram calories needed for evaporating 1 g of water, or as the amount of heat needed for producing 1 g of water vapor of the same temperature as the water. Only in the latter form is the definition applicable to sea water. The latent heat of evaporation of sea water has not been examined, but it is generally assumed that the difference between that and pure water is insignificant; therefore, between temperatures of 0° and 30°C, the formula
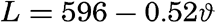
Adiabatic Temperature Changes. When a fluid is compressed, without gain or loss of heat to the surroundings, work is performed on the system and there is a rise in temperature. Conversely, if expansion takes place, the liquid itself gives up energy, which is reflected in a drop in temperature. Such adiabatic temperature changes are well known and important in the atmosphere. Sea water is compressible, and the effects of adiabatic processes, although small, must be taken into account when studying the vertical distribution of temperature in the great depths of the oceans and in deep isolated basins where the adiabatic heating may lead to a temperature increase toward the bottom (for example, p. 739). Adiabatic cooling is of immediate practical concern when water samples are taken with thermally insulated water bottles and the temperature of the water sample is determined after it has been raised to the surface (p. 355)
| Depth (m) | Temperature (°C) | ||||||||
|---|---|---|---|---|---|---|---|---|---|
| −2 | 0 | 2 | 4 | 6 | 8 | 10 | 15 | 20 | |
| 0. | 0.016 | 0.035 | 0.053 | 0.078 | 0.087 | 0.103 | 0.118 | 0.155 | 0.190 |
| 1,000. | 0.036 | 0.054 | 0.071 | 0.087 | 0.103 | 0.118 | 0.132 | 0.166 | 0.199 |
| 2,000. | 0.056 | 0.073 | 0.089 | 0.104 | 0.118 | 0.132 | 0.146 | 0.177 | 0.207 |
| 3,000. | 0.075 | 0.091 | 0.106 | 0.120 | 0.133 | 0.146 | 0.159 | 0.188 | |
| 4,000. | 0.093 | 0.108 | 0.122 | 0.135 | 0.147 | 0.159 | 0.170 | 0.197 | |
| 5,000. | 0.110 | 0.124 | 0.137 | 0.149 | |||||
| 6,000. | 0.120 | 0.140 | 0.152 | 0.163 | |||||
| 7,000. | …… | 0.155 | 0.165 | 0.175 | |||||
| 8,000. | …… | 0.169 | 0.178 | 0.187 | |||||
| 9,000. | …… | 0.182 | 0.191 | 0.198 | |||||
| 10,000. | …… | 0.194 | 0.202 | 0.209 | |||||
Any adiabatic effect is related to changes in pressure, but in the sea the pressure can be considered proportional to the depth, and adiabatic temperature changes can be given as changes per unit of depth instead of per unit of pressure. According to Lord Kelvin, the change in temperature for each centimeter of vertical displacement is
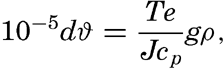
| Depth (m) | Temperature, ϑm (°C) | ||||||||||||
|---|---|---|---|---|---|---|---|---|---|---|---|---|---|
| − 2 | −1 | 0 | 1 | 2 | 3 | 4 | 5 | 6 | 7 | 8 | 9 | 10 | |
| 1,000…… | 2.6 | 3.5 | 4.4 | 5.3 | 6.2 | 7.0 | 7.8 | 8.6 | 9.5 | 10.2 | 11.0 | 11.7 | 12.4 |
| 2,000…… | 7.2 | 8.9 | 10.7 | 12.4 | 14.1 | 15.7 | 17.2 | 18.8 | 20.4 | 21.9 | 23.3 | 24.8 | 26.2 |
| 3,000…… | 13.6 | 16.1 | 18.7 | 21.2 | 23.6 | 25.9 | 28.2 | 30.5 | 32.7 | 34.9 | 37.1 | 39.2 | 41.2 |
| 4,000…… | 21.7 | 25.0 | 28.4 | 31.6 | 34.7 | 37.7 | 40.6 | 43.5 | 46.3 | 49.1 | 51.9 | 54.6 | 57.2 |
| 5,000…… | 31.5 | 35.5 | 39.6 | 43.4 | 47.2 | 50.9 | 54.4 | ||||||
| 6,000…… | 42.8 | 47.5 | 52.2 | 56.7 | 61.1 | 65.3 | 69.4 | ||||||
| 7,000…… | …… | …… | 66.2 | 71.3 | 76.2 | 80.9 | 85.5 | ||||||
| 8,000…… | …… | …… | 81.5 | 87.1 | 92.5 | 97.7 | 102.7 | ||||||
| 9,000…… | …… | …… | 98.1 | 104.1 | 109.9 | 115.6 | 121.0 | ||||||
| 10,000…… | 115.7 | 122.1 | 128.3 | 134.4 | 140.2 | ||||||||
The temperature that a water sample would attain if raised adiabatically to the sea surface has been called the potential temperature (Helland-Hansen, 1912b) and has been designated Θ. Thus, Θ = ϑm − Δϑ, where ϑm, is the temperature in situ and Δϑ is the amount by which the temperature would decrease adiabatically if the sample were raised to the surface. The potential temperature can be obtained from a table of the adiabatic gradients by step-wise computations. Such computations are long and tedious, but Helland-Hansen (1930) has prepared a, convenient table for Δϑ which has been reproduced in table 12. The table is based on a salinity of 34.85 ‰ (σ0 = 28.0) and is applicable in general to the deep ocean areas, because in these the salinity does not differ much from 34.85 ‰ and because the effect of salinity on the adiabatic processes is small. It may be seen that if water of 2°C is raised adiabatically from 8000 m to the surface, Δϑ = 0.925°, and therefore the potential temperature of that water is 1.075°. The adiabatic cooling of water of different