Section 3

Of the motion of bodies in eccentric conic sections .

Proposition 11—
Problem 6

Let a body be revolved on an ellipse; there is required the law of centripetal force being directed to a focus of the ellipse . [See fig. A.16.]

Let S be a focus of the ellipse above. Let SP be drawn cutting not only the diameter of the ellipse DK at E , but also the ordinate QV in X , and let the parallelogram QXPR be completed.

It is clear the EP is equal to the semi-major axis AC , seeing that, when from the other focus H of the ellipse the line HI has been drawn parallel to CE (because CS and CH are equal), ES and EI would be equal, and hence EP would be half the sum of PS and PI , that is (because HI and PR are parallel, and the angles IPR and HPZ are equal), of PS and PH which are conjointly equal to the total axis 2AC .

Let the perpendicular QT be dropped to SP , and, after calling the principal latus rectum (or 2BC² / AC ) of the ellipse L ,

there will be L × QR to L × PV as QR to PV , that is, as PE (or AC ) to PC ;
and L × PV to GV × VP as L to GV ;
and GV × VP to QV² as CP² to CD² ;
and (by Lemma 8) QV² to QX² , is, with points P and Q coalescing, a ratio of equality;
and QX² or QV² is to QT² as EP² to PF² , that is, as CA² to PF² , or (by Lemma 12) as CD² to CB² .

And when all these ratios are combined,

L × QR becomes to QT² as (AC to PC ) × (L to GV ) × (CP² to CD² ) × (CD² to CB² )
that is, as AC × L (or 2CB² ) × CP² becomes to PC × GV × CB² ,
that is, as 2PC to GV .

But with the points Q and P coalescing, 2PC and GV are equal. Therefore the proportionals of these, L × QR and QT ² , are also equal. Let these equals be multiplied by SP² / QR , and L × SP² will be made equal to SP² × QT² / QR .

Therefore (by the corollary of Theorem 5) the centripetal force is reciprocally as L × SP² , that is, reciprocally in the doubled ratio of the distance SP . Which was to be found.

With the same brevity with which we transferred the fifth Problem to the parabola and the hyperbola, it would be possible to do the same here;

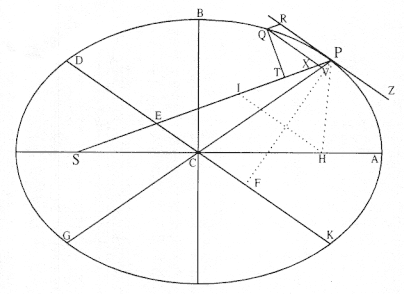
Figure A.16
Proposition 11.

but because of the dignity of the problem and its use in what follows, it will be no trouble to corroborate the other cases by [separate] proof.

Proposition 12—
Problem 7

Let a body be moved on a hyperbola; there is required the law of centripetal force being directed to a focus of the figure . [See fig. A.17.]

Let CA and CB be semi-axes of the hyperbola; PG and KD conjugate diameters; PF and QT perpendiculars to the diameters; and QV ordinate to the diameter GP . Let SP be drawn cutting both the diameter DK at E and the ordinate QV at X , and let the parallelogram QRPX be completed.

It is clear that EP is equal to the transverse semi-major axis AC , seeing that, when from the other focus H of the hyperbola the line HI has been drawn parallel to CE (because CS and CH are equal), ES and EI would be equal, and hence EP would be the half-difference of PS and PI , that is (because HI and PR are parallel, and the angles IPR and HPZ are equal), of PS and PH , whose difference is equal to the total axis 2AC .

Let the perpendicular QT be dropped to SP , and, after calling the principal latus rectum (or 2BC² / AC ) of the hyperbola L ,

there will be L × QR to L × PV as QR to PV ; that is, as PE (or AC ) to PC ;
and L × PV to GV × VP as L to GV ;

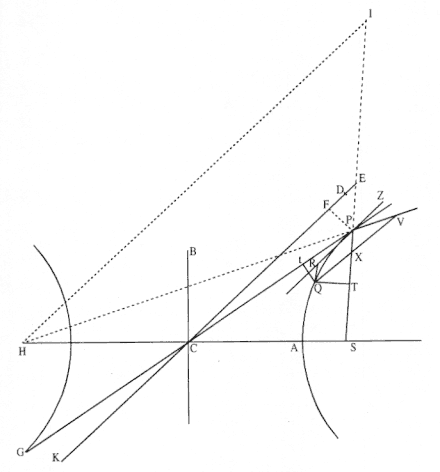
Figure A.17
Proposition 12.

and GV × VP to QV² as CP² to CD² ;
and (by Lemma 8) QV² to QX² , becomes, with points P and Q coalescing, a ratio of equality;
and QX² or QV² is to QT² as EP² to PF² , that is, as CA² to PF² , or (by Lemma 12) as CD² to CB² .

And when all these ratios are combined,

L × QR becomes to QT² as (AC to PC ) × (L to GV ) × (CP² to CD² ) × (CD² to CB² ),
that is, as AC × L (or 2 CB² ) × PC² becomes to PC × GV × CB² ,
that is, as 2PC to GV .

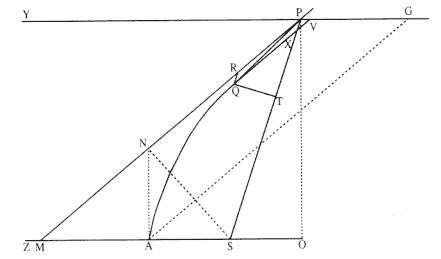
Figure A.18
Lemma 14.

But with points Q and P coalescing, 2PC and GV are equal. Therefore the proportionals to these, L × QR and QT² , are also equal. Let these equals be multiplied by SP² / QR , and L × SP² will be made equal to SP² × QT² / QR .

Therefore (by the corollary of Theorem 5) the centripetal force is reciprocally as L × SP² , that is, reciprocally in the doubled ratio of the distance SP . Which was to be found.

In the same manner it is proven that a body, with this centripetal force changed to centrifugal, will be moved in a conjugate hyperbola.

Lemma 13

The latus rectum of a parabola pertaining to any vertex is quadruple the distance of that vertex from the focus of the figure .

This is evident from the Conics .

Lemma 14

A perpendicular which is dropped from the focus of a parabola to its tangent is a mean proportional between the distances of the focus from the point of contact and from the principal vertex of the figure . [See fig. A.18.]

For let APQ be the parabola, S its focus, A the principal vertex, P the point of contact, PO the ordinate to the principal diameter, PM a tangent meeting the principal diameter at M , and SN a perpendicular line from the focus to the tangent. Let AN be joined, and, because MS and SP, MN and NP, MA and AO are equals, straight lines AN and OP will be parallel,

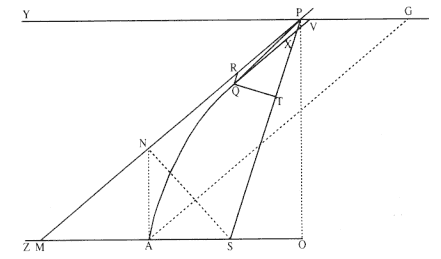
Figure A.19
Proposition 13.

and hence the triangle SAN will have a right angle at A and likewise for the equal triangles SMN and SPN . Therefore PS is to SN as SN to SA . Which was to be proven.

Corollary 1. PS² is to SN² as PS is to SA .

Corollary 2 . And, because SA is given, SN² is as PS .

Corollary 3 . And the meeting of any tangent PM with the straight line SN , which is perpendicular from the focus to it, falls on the straight line AN , which touches the parabola on the principal vertex.

Proposition 13—
Problem 8

Let a body be moved on the perimeter of a parabola; there is required the law of centripetal force being directed to the focus of this figure . [See fig. A.19.]

Let the construction of Lemma 14 remain, and let P be the body on the perimeter of the parabola, and then, from the position Q to which the body next moves, draw QR parallel to SP and QT perpendicular, and also QV parallel to the tangent and meeting both the diameter YPG at V and the distance SP at X .

Now because triangles PXV and MSP are similar, and the sides SM and SP of one are equal, the sides PX or QR and PV of the other are equal. But, from the Conics , the square of the ordinate QV is equal to the rectangle [generated] by the latus rectum and the segment PV of the diameter, that is (by Lemma 13), equal to the rectangle 4PS × PV or 4PS × QR ; and, with points P and Q coalescing, the ratio QV to QX (by Lemma 8) is one of equality.

Therefore in this case QX ² is equal to the rectangle 4PS × QR . But (because angles QXT, MPS , and PMO are equal), QX² is to QT² as PS² to SN² , that is (by Corollary 1, Lemma 14), as PS to AS , that is, as 4PS × QR to 4AS × QR , and therefore (by Proposition 9, Book 5 Elements ), QT² and 4AS × QR are equal.

Let these equals be multiplied by SP² / QR and SP² × QT² / QR will become equal to SP² × 4AS ; and therefore (by the corollary of Theorem 5) the centripetal force is reciprocally as SP² × 4AS , that is, because 4AS is given, reciprocally in the doubled ratio of the distance SP . Which was to be found.

Corollary 1 . From the last three propositions it follows that if any body P should depart from position P along any straight line PR , with any velocity, and is at the same time acted upon by a centripetal force that is reciprocally proportional to the square of the distance from the center, this body will be moved in one of the sections of conics having a focus at the center of forces; and conversely.

Corollary 2 . And if the velocity with which the body departs from its position P should be such that the line element PR could be described in some minimal particle of time, and the centripetal force should be able to move the same body through the space QR in the same time; this body will be moved in some conic section whose latus rectum is that quantity QT² / QR , which would ultimately result when the line segments PR and QR are diminished infinitely. I place the circle [in the same category] with the ellipse in these corollaries, but I exclude the case where the body descends straight to the center.

Proposition 14—
Theorem 6

If several bodies should be revolved around a common center, and [if ] the centripetal force should decrease in the doubled ratio of the distances from the center, [then ] I say that the latera recta of orbits are in the doubled ratio of the areas that bodies describe by radii constructed to the center in the same time .

For (by Corollary 2, Problem 8) the latus rectum L is equal to the quantity QT² / QR , which would ultimately result when points P and Q coalesce. But the very small line QR , given the time, is as the generating centripetal force, that is (by hypothesis), reciprocally as SP ² . Therefore QT² / QR is as QT² × SP² ; that is, the latus rectum L in the doubled ratio of the area QT × SP . Which was to be proven.

Corollary . Hence the total area of the ellipse, and, proportional to it, the rectangle [generated] by the axes, is in the ratio compounded of the halved ratio of the latus rectum and the whole ratio of the periodic time.

Proposition 15—
Theorem 7

With the same suppositions, I say that the periodic times in ellipses are in the three-halved ratio of the transverse axes .

For the minor axis is the mean proportional between the major axis (which I call transverse) and the latus rectum , and therefore the rectangle [generated] by the axes is in a ratio compounded of the halved ratio of the latus rectum and the three-halved ratio of the transverse axis. But this rectangle, by the corollary of the sixth theorem, is in a ratio compounded of the halved ratio of the latus rectum and the whole ratio of the periodic time. Let the halved ratio of the latus rectum be taken away from each side and there will remain the three-halved ratio of the transverse axis equal to the ratio of the periodic time. Which was to be proven.

Corollary . The periodic times in ellipses, therefore, are the same as in circles whose diameters are equal to the major axes of ellipses.

Proposition 16—
Theorem 8

With the same suppositions, and with straight lines drawn to bodies that touch the orbits in the same places, and with perpendiculars dropped to these tangents from a common focus, I say that the velocities of the bodies are in a ratio compounded of the ratio of perpendiculars inversely, and the half ratio of the latera recta directly . [See fig. A.20.]

From the focus S to the tangent PR drop the perpendicular SY , and the velocity of the body P will be reciprocally in the halved ratio of the quantity SY² / L . For that velocity is as the smallest possible arc PQ described in a given particle of time, that is (by Lemma 7), as the tangent PR , that is (because PR is proportional to QT as SP to SY ), as (SP × QT ) / SY , or as SY reciprocally and SP × QT directly; and there is SP × QT as the area described in a given time, that is (by Theorem 6), in the halved ratio of the latus rectum . Which was to be proven.

Corollary 1 . The latera recta are in a ratio compounded of the doubled ratio of the perpendiculars and the doubled ratio of the velocities.

Corollary 2 . The velocities of bodies at the greatest and smallest distances from the common focus are in a ratio compounded of the ratio of distances inversely and the halved ratio of the latera recta directly. For the perpendiculars are now those very distances.

Corollary 3 . Thus the velocity in a conic section, at the smallest distance from the focus, is to the velocity in a circle at the same distance from the center, in the halved ratio of the latus rectum to that distance doubled.

Corollary 4 . The velocities of bodies orbiting on ellipses at mean distances from the common focus are the same as those of bodies orbiting

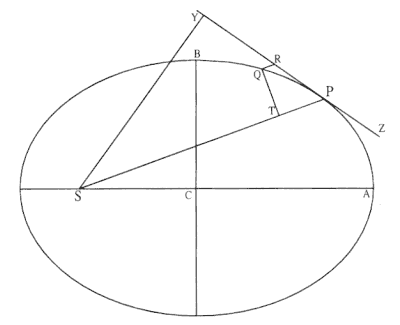
Figure A.20
Proposition 16.

on circles at the same distances, that is (by Corollary 6, Theorem 4), reciprocally in the halved ratio of the distances. For the perpendiculars are now [equal to] the semi-minor axes, and these are as the mean proportionals between the distances and the latera recta . Let this ratio be compound inversely with the halved ratio of the latera recta directly, and it will become the half ratio of the distances inversely.

Corollary 5 . In the same [figure] or in equal figures, or even in unequal figures, whose latera recta are equal, the velocity of a body is reciprocally as the perpendicular dropped from the focus to the tangent.

Corollary 6 . In a parabola the velocity is reciprocally in the halved ratio of the distance of the body from the focus of the figure; in the ellipse it is less than in this ratio, in the hyperbola greater. For (by Corollary 2, Lemma 14) the perpendicular dropped from the focus to the tangent of a parabola is in the halved ratio of the distance.

Corollary 7 . In a parabola the velocity is everywhere to the velocity of a body orbiting on a circle at the same distance in the halved ratio of the number 2 to unity; in the ellipse it is less than in this ratio, in the hyperbola greater. For by the second corollary of this [proposition], the velocity on the vertex of a parabola is in this ratio, and by the sixth corollary of

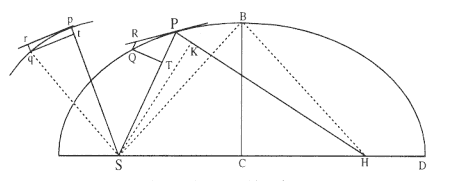
Figure A.21
Proposition 17.

this [proposition] and of the fourth theorem, the same proportion is preserved at all distances. Hence also in a parabola the velocity everywhere is equal to the velocity of a body revolving in a circle at half the distance; in the ellipse it is less, in the hyperbola greater.

Corollary 8 . The velocity of [a body] orbiting on any conic section is to the velocity of [a body] orbiting on a circle at a distance of half of the latus rectum of the section, as that distance to the perpendicular dropped from the focus to the tangent of the section. It is evident by the fifth corollary.

Corollary 9 . From this (by Corollary 6, Theorem 4) since the velocity of [a body] orbiting on this circle is to the velocity of [a body] orbiting in any other circle reciprocally in the halved ratio of the distances, similarly the velocity of [a body] orbiting on a conic section will become to the velocity of [a body] orbiting on a circle at the same distance, as the mean proportional between that common distance and half the latus rectum of the section to the perpendicular dropped from the common focus to the tangent of the section.

Proposition 17—
Problem 9

Supposing that the centripetal force be made reciprocally proportional to the square of the distance from its center, and that the absolute quantity of that force is known; there is required a line which a body will describe, when leaving from a given position with a given velocity along a given straight line . [See fig. A.21.]

Let the centripetal force being directed to point S be that which makes body p orbit in any given orbit pq , and let the velocity of this [body] at position p be known. Let the body P be released from the position P with a given velocity along line PR , and soon after let it deflect under the compulsion of the centripetal force into the conic section PQ . The straight line PR , therefore, will touch this at P .

In the same way let another straight line pr touch the orbit pq at p , and if from S perpendiculars are understood to be dropped to those tangents, there will be (by Corollary 1, [Proposition 16], Theorem 8) the latus rectum of the conic section to the latus rectum of the given orbit in a ratio compounded of the doubled ratio of the perpendiculars and the doubled ratio of the velocities, and therefore [the latus rectum of the conic section] is given. Let that be L .

In addition the focus S of the conic section is given. Let angle RPH be the complement of angle RPS to two right angles, and there will be given in position the line PH in which the other focus H is located. After the perpendicular SK has been dropped to PH , and the conjugate semi-axis BC has been erected, there is

To each side let 2KP x PH + L x (SP + PH ) - SP² - PH² be added and there will come L x (SP + PH ) = 2SP x PH + 2KP x PH , or, SP + PH to PH as 2SP + 2KP to L .

Hence PH is given in length as well as position.

Specifically, if the velocity of the body at P should be such that the latus rectum L would be less than (2SP + 2KP ), [then] PH will lie on the same part of the tangent PR with the line PS ; and thus the figure will be an ellipse, and it will be given both from the given foci S and H and from the [given] principal axis (SP + PH ).

But if the velocity of the body is so great that the latus rectum L is equal to (2SP + 2KP ), [then] the length of PH will be infinite, and as a result the figure will be a parabola having its axis SH parallel to the line PK , and from this it will be given.

But if the body is released from its position P with an even greater velocity, [then] the length PH must be taken on the other side of the tangent, and thus, with the tangent proceeding between the foci, the figure will be a hyperbola having its principal axis equal to the difference of the lines SP and PH , and from this it will be given. Which was to be found.

Corollary 1 . Hence in every conic section, given the principal vertex D , the latus rectum L , and the focus S ; the other focus H is given by taking DH to DS as the latus rectum is to the difference between the latus rectum and 4DS . For the proportion SP + PH to PH as 2SP to L , in the case of this corollary it would be DS + DH to DH as 4DS to L , and, by dividing, DS to DH as 4DS – L to L .

Corollary 2 . From there if the velocity of a body is given at its principal vertex D , the orbit will be found expeditiously, to be sure by taking its latus rectum to twice the distance DS in the doubled ratio of this given velocity to the velocity of the body revolving on a circle at the distance DS (by Corollary 3, Theorem 8), and then DH to DS as the latus rectum to the difference between the latus rectum and 4DS .

Corollary 3 . Hence even if a body should be moved on any conic section, and should be disturbed from its orbit by any impulse, the orbit can be ascertained in which it would afterward continue its course. For by combining the particular motion of the body with that motion which the impulse alone would generate, there will be obtained the motion with which the body, from the given place of the impulse, will depart from its position along a given straight line.

Corollary 4 . And if that body, pressed by some outside force, is continually perturbed, its course will become known as nearly as possible by collecting the changes which that force induces at certain points and by estimating from an analogy of the sequence the continual changes at intermediate positions.