12.1—
Introduction

The first theory of metabolic control was introduced into plant physiology in 1905 by F. F. Blackman and has become known as the 'Law of Limiting Factors' which states that 'when a process is conditioned as to its rapidity by a number of separate factors the rate of the process is limited by the pace of the slowest reaction'.

It is not possible to derive a rate equation from this statement and discussions of the 'Law' tend to be by analogy, e.g. the strength of a chain is the strength of its weakest link. Experimentally, the statement has led to the fruitless search for metabolic master reactions. Mathematically, we can derive rate equations for multistep reactions and establish that every step contributes to the overall rate. Theoretically Blackman's statement is a denial of the steady state; if a metabolic sequence is in a steady state then the concentration of each intermediate is constant and the individual reactions proceed at the same rate—hence no reaction can be described as the slowest.

12.1.1—
Pacemakers

If in a metabolic sequence all reactions are proceeding at the same pace then a single reaction may be almost entirely responsible for determining that pace; such a reaction has been called a 'bottleneck' or pacemaker and much work in metabolic control is directed towards the identification of such control points. Krebs and Kornberg (1957) in a consideration of pacemakers stated 'there is a principle which may guide the search for pacemakers. As pacemakers are reactions of variable rate, the level of substrate concentration of the pacemaker must vary inversely with the rate: it must increase when the reaction rate decreases'. It should be possible to take published data for a metabolic pathway, apply the Krebs-Kornberg principle and so determine which reaction is the pacemaker. It turns out that in some cases the Krebs-Kornberg principle cannot be applied. For example in some tissues when the rate of glycolysis is increased not a single compound shows the expected reduction in concentration.

12.1.2—
Occam's Razor

William of Occam, the mediaeval controversialist, formulated a procedural rule, that when a number of possible solutions can be proposed for a problem,

one should accept the simplest solution until it is shown to be untenable. The Krebs-Kornberg principle is a simple solution to a complex problem and in certain cases it yields a valid solution. However, when it is not applicable we must examine more complex solutions.

12.1.3—
Systems Properties

The rate equation for a metabolic pathway includes parameters for all the enzymes and variables for all the metabolites (Waley, 1964). The flux through the pathway is a systemic property in which all the parameters interact as a system. If a single parameter is altered, say the activity of a single enzyme, then the whole system responds and adjusts to that change. The extent to which an enzyme can be considered a control step is the extent to which a fractional change in its activity produces a fractional change in the flux through the system. The ratio

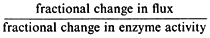
has been termed the sensitivity coefficient for that step (Kacser & Burns, 1973). If a 1 % change in activity of an enzyme produces a 1% change in flux through the system, then the sensitivity coefficient is 1 and the enzyme must be fully controlling the flux and is a pacemaker. However, the sum of all the sensitivity coefficients for a metabolic sequence is equal to unity and only in special circumstances can a single pacemaker be identified. That the sensitivity co-efficient of a particular enzyme is a system property, only in part determined by its own parameters, can be intuitively understood by an example. In a given situation an enzyme may have a very small sensitivity coefficient and contribute little to the overall control of the system. If however the activity of this enzyme is drastically reduced its sensitivity coefficient may be increased and it may contribute significantly to the overall flux. Since the sum of sensitivity coefficients must always equal unit the sensitivity coefficients of all the other enzymes must have changed despite the fact that their parameters are unchanged.

Much work on metabolic control is concerned with the identification of control points. Since each reaction contributes to the overall control a sense of judgement is necessary to identify major control points and it must be remembered that control may pass from one reaction to another as the flux through the system changes.